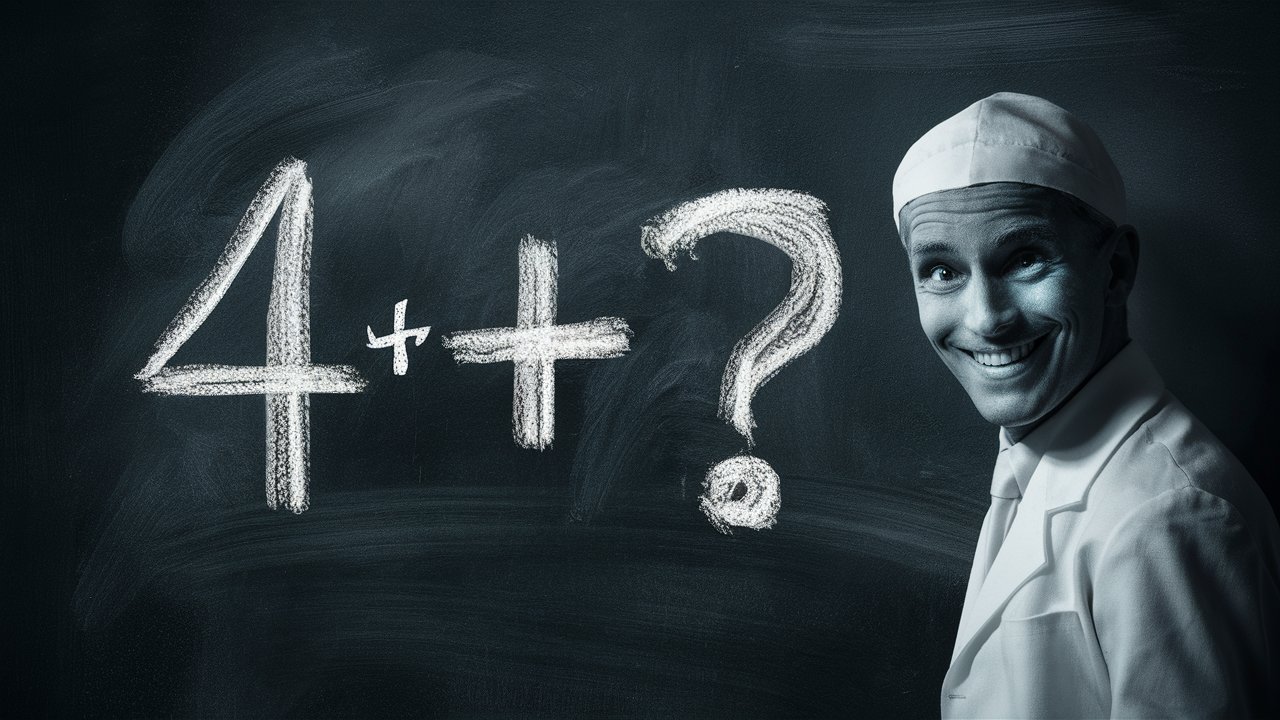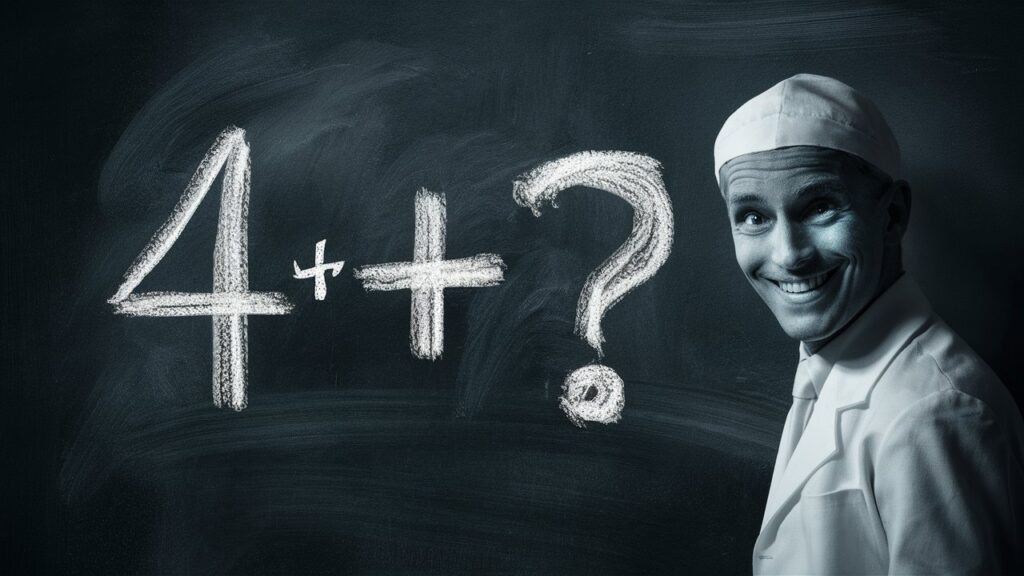
Understanding the Concept of “What Expansion Pairs Truth 4+3″Addition is one of the foundational number juggling operations that everybody experiences early in their instruction. Among the numerous expansion procedures, the “pairs truth” stands out as a especially valuable apparatus. In this article, we will dive into the concept of expansion copies actualities, with a specific center on understanding what expansion copies reality relates to the whole of 4+3. We will investigate the significance of these realities in science, how they can be connected, and give answers to a few regularly inquired questions.
What Are Expansion Copies Facts?
Addition copies actualities are basic math conditions where a number is included to itself. For occurrence, the copies actualities for the numbers 1 through 10 are:
1 + 1 = 2
2 + 2 = 4
3 + 3 = 6
4 + 4 = 8
5 + 5 = 10
6 + 6 = 12
7 + 7 = 14
8 + 8 = 16
9 + 9 = 18
10 + 10 = 20
These truths are called “pairs” since the same number is utilized twice. They are basic in the early stages of learning expansion since they offer assistance construct a solid establishment for more complex number juggling operations.
The Significance of Pairs Truths in Mathematics
Understanding and memorizing pairs truths is significant for a few reasons:
Building Piece for More Complex Calculations: Copies actualities serve as a venturing stone for learning other expansion techniques, such as close copies and copies also one.
Enhancing Mental Math Aptitudes: By acing these essential truths, understudies can perform calculations more rapidly and precisely in their heads, which is an fundamental ability for higher-level math.
Boosting Certainty: Knowing these actualities gives understudies a sense of achievement and certainty, which empowers them to handle more challenging scientific problems.
Relating Copies Realities to 4+3
Now, let’s address the particular address: What expansion pairs truth relates to the entirety of 4+3?
When we see at the entirety 4+3, it is not quickly a copies truth since the numbers being included are not the same. Be that as it may, understanding pairs truths can still offer assistance us unravel this issue more efficiently.
Near Copies Strategy
One successful way to approach this issue is by utilizing the “close pairs” technique. This includes finding the closest copies reality and altering the result in like manner. For 4+3, the closest copies actualities are:
4 + 4 = 8
3 + 3 = 6
Since 4 is one more than 3, we can utilize the reality that 4 + 4 = 8 and at that point subtract 1 to discover the entirety of 4+3. This is since we are basically including one less than the twofold of 4. Therefore:
4 + 3 = (4 + 4) – 1 = 8 – 1 = 7
Alternatively, we can utilize the pairs truth for 3:
3 + 3 = 6
Since 4 is one more than 3, we include 1 to the sum:
4 + 3 = (3 + 3) + 1 = 6 + 1 = 7
Visual Representation
To encourage outline this, consider the visual representation of copies realities and close doubles:
4 + 4: Envision two bunches of 4 objects each. When combined, they make 8 objects.
4 + 3: Envision one gather of 4 objects and another gather of 3 objects. When combined, they make 7 objects, which is one less than the 8 objects from the 4 + 4 pairs fact.
Practical Applications of Pairs Facts
Understanding pairs and close pairs truths is not fair an scholarly work out; it has viable applications in ordinary life and more progressed science. Here are a few examples:
Everyday Applications
Shopping: When calculating the add up to taken a toll of different things, knowing copies can speed up the prepare. For illustration, if each apple costs $3 and you purchase 2, you can rapidly calculate the taken a toll as 3 + 3 = 6.
Cooking: When multiplying a formula, knowing that multiplying 4 mugs of flour implies 8 mugs can spare time and exertion in calculations.
Advanced Numerical Concepts
Algebra: In variable based math, understanding fundamental expansion truths makes a difference in fathoming conditions more proficiently. For occurrence, knowing that 2x = 8 leads to x = 4 is a coordinate application of doubles.
Geometry: When calculating regions and borders, copies realities can disentangle the prepare. For case, the border of a square with side length 4 is 4 + 4 + 4 + 4 = 16, which is basically 4 multiplied twice.
Frequently Inquired Questions (FAQ)
What Are Pairs Facts?
Doubles truths are basic expansion conditions where a number is included to itself. They are essential in learning number juggling and building a establishment for more complex math.
How Do Pairs Truths Offer assistance in Learning Math?
Doubles realities offer assistance in learning math by giving a speedy and simple way to perform essential expansion, which serves as a building piece for more complex calculations. They upgrade mental math abilities and boost confidence.
What Is a Close Pairs Strategy?
A close copies methodology includes utilizing the closest pairs truth to unravel an expansion issue. For illustration, to illuminate 4+3, you can utilize the pairs truth 4+4 and at that point subtract 1.
Can Copies Realities Be Utilized in Genuine Life?
Yes, copies realities have commonsense applications in regular life, such as calculating costs whereas shopping, multiplying formulas, and fathoming more complex scientific issues in polynomial math and geometry.
Why Is It Imperative to Memorize Pairs Facts?
Memorizing pairs truths is vital since it makes a difference construct a solid establishment for number juggling, improves mental math abilities, and makes tackling more complex issues simpler and faster.
Conclusion
Understanding and utilizing expansion pairs truths is a principal perspective of science instruction. Whereas 4+3 is not a pairs truth, the close pairs procedure gives an productive way to fathom it by relating it to the closest pairs truths. By acing these fundamental concepts, understudies can improve their scientific aptitudes and certainty, making them way better arranged for more progressed numerical challenges. Whether in ordinary circumstances or higher-level math, the information of copies realities demonstrates to be an priceless instrument.